Article
Version 1
Preserved in Portico This version is not peer-reviewed
Extrinsic Quaternion Spin
Version 1
: Received: 1 February 2023 / Approved: 3 February 2023 / Online: 3 February 2023 (03:51:33 CET)
Version 2 : Received: 31 March 2023 / Approved: 3 April 2023 / Online: 3 April 2023 (03:01:35 CEST)
Version 3 : Received: 26 January 2024 / Approved: 29 January 2024 / Online: 29 January 2024 (08:47:38 CET)
Version 4 : Received: 18 May 2024 / Approved: 20 May 2024 / Online: 20 May 2024 (12:37:38 CEST)
Version 5 : Received: 17 June 2024 / Approved: 18 June 2024 / Online: 18 June 2024 (08:15:32 CEST)
Version 2 : Received: 31 March 2023 / Approved: 3 April 2023 / Online: 3 April 2023 (03:01:35 CEST)
Version 3 : Received: 26 January 2024 / Approved: 29 January 2024 / Online: 29 January 2024 (08:47:38 CET)
Version 4 : Received: 18 May 2024 / Approved: 20 May 2024 / Online: 20 May 2024 (12:37:38 CEST)
Version 5 : Received: 17 June 2024 / Approved: 18 June 2024 / Online: 18 June 2024 (08:15:32 CEST)
A peer-reviewed article of this Preprint also exists.
Sanctuary, B. Quaternion Spin. Mathematics 2024, 12, 1962. https://doi.org/10.3390/math12131962 Sanctuary, B. Quaternion Spin. Mathematics 2024, 12, 1962. https://doi.org/10.3390/math12131962
Abstract
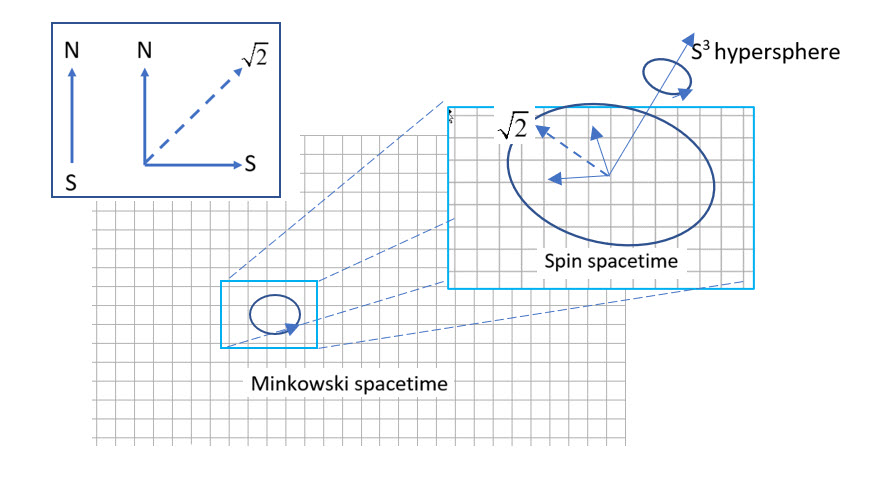
We present an analysis of the Dirac equation when the spin symmetry is changed from SU(2) to the quaternion group, $Q_8$, afforded by multiplying one of the $\gamma$-matrices by the imaginary number $i$. The resulting spin equation separates into distinct and complementary Hermitian and antiHermitian spaces. The former describes a 2D structured spin and the latter its helicity, generated by a unit quaternion. We discuss some consequences.
Keywords
foundations of physics; Dirac equation; spin; quantum theory; non-locality; helicity
Subject
Physical Sciences, Theoretical Physics
Copyright: This is an open access article distributed under the Creative Commons Attribution License which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Comments (2)
We encourage comments and feedback from a broad range of readers. See criteria for comments and our Diversity statement.
Leave a public commentSend a private comment to the author(s)
* All users must log in before leaving a comment









Commenter: Mark Hadley
The commenter has declared there is no conflict of interests.
What we can say for sure is that a difference representation of the gamma matrices gives no new physics.
However using matrices that do not satisfy the Clifford algebra might seem like a small and acceptable change to the author, but it is not. The resulting equation simply won't work. It won't transform correctly under spacetime symmetries and you won't be able to define a conserved particle number.
It is not worth proceeding further into the detail of the paper when it is based on equations that cannot represent real particles.
If the author wants to change the Dirac equation he first needs to show in detail that 1) all important results are still valid 2) if it is valid then the next step is to show that it is physically different.
Commenter:
The commenter has declared there is no conflict of interests.
The only change I made is to multiply one of the well known gamma matrices by the imaginary number "i". From that small change the rest follows.
In the last two points, Hadley ignores the fact that I show the resulting quaternion equation does indeed recover the original Dirac equation. The new result is quaternion spin which reveals properties that formulate coherence This is new and its implication appear to have far reaching consequences for our understanding of the foundation of physics