An Analysis of Prize Money Distributions in Snooker

Wim Hordijk

doi:10.20944/preprints202306.1532.v2

Preprint Article Version 2 Preserved in Portico This version is not peer-reviewed

Version 1 : Received: 20 June 2023 / Approved: 21 June 2023 / Online: 21 June 2023 (10:57:53 CEST)
Version 2 : Received: 15 January 2024 / Approved: 15 January 2024 / Online: 15 January 2024 (13:08:02 CET)

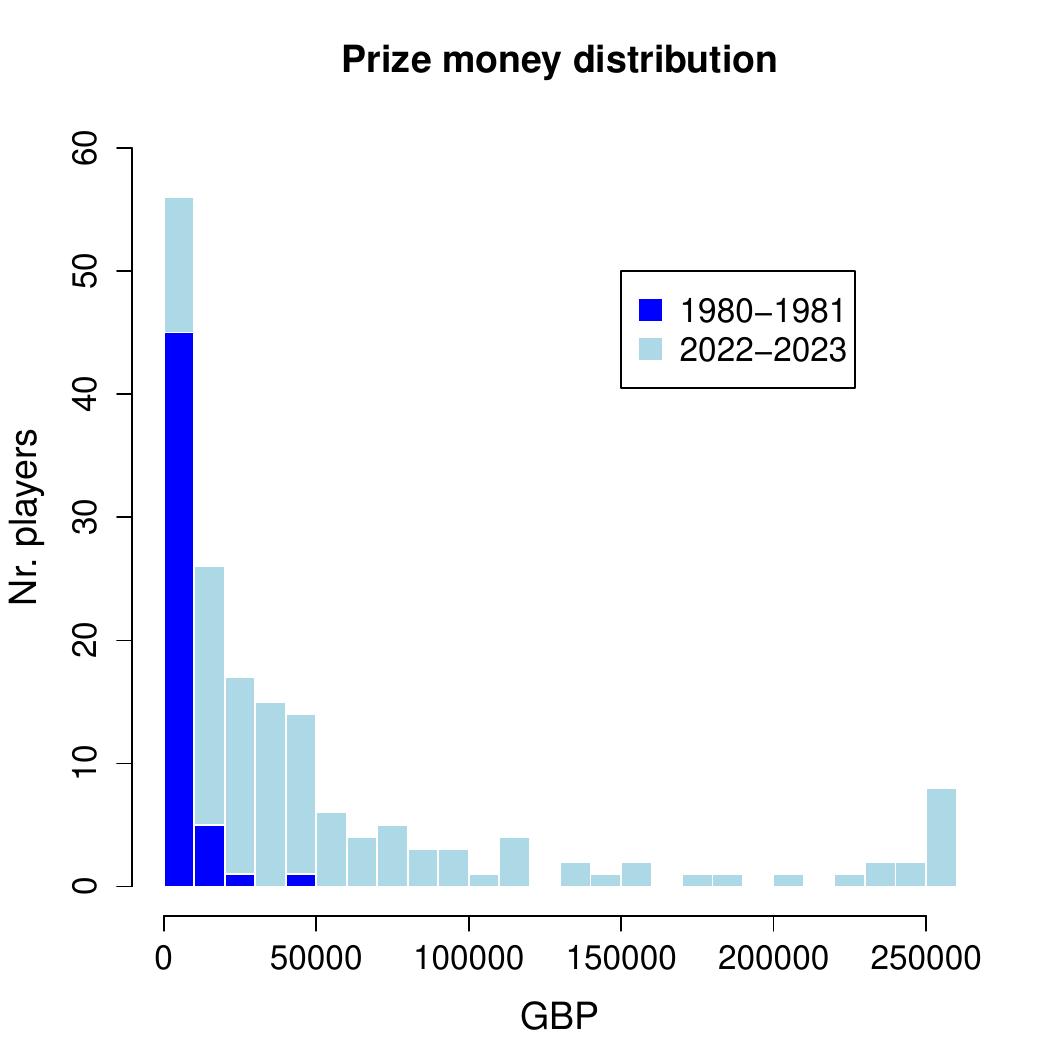
Four decades of snooker rankings are analyzed in terms of prize money distributions. In particular, it is investigated how much these rankings are dominated by the top players, and also by players from the UK. The main conclusions are: (1) with an increasing amount of available prize money, the rankings are less dominated by the top players, and (2) players from the UK have historically earned a significantly larger share of the available prize money than could be reasonably expected based on the actual percentage of UK players.

snooker; rankings; prize money; pareto principle

Social Sciences, Tourism, Leisure, Sport and Hospitality

Comment 1

Received: 15 January 2024
Commenter: Wim Hordijk
Commenter's Conflict of Interests: Author

Comment: This version contains a more detailed and clear explanation of the statistical methods that are used.

+ Respond to this comment

Not displayed online.

Please leave your comment to this article below.

Mathematical equations can be typed in either LaTeX formats \\[ ... \\] or $$ ... $$, or MathML format <math> ... </math>. Try the LaTeX or MathML example.

Type equation:

Preview:

Copy equation into message below

Close menu

Please click a symbol to insert it into the message box below:

Please enter the link here:

Optionally, you can enter text that should appear as linked text:

Copy link into message below

Close menu

Please enter or paste the URL to the image here (please only use links to jpg/jpeg, png and gif images):

Copy image into message below

Close menu

Type author name or keywords to filter the list of references in this group (you can add a new citation under Bibliography):

No existing citations in Discussion Group

Wikify editor is a simple editor for wiki-style mark-up. It was written by MDPI for Sciforum in 2014. The rendering of the mark-up is based on Wiky.php with some tweaks. Rendering of mathematical equations is done with MathJax. Please send us a message for support or for reporting bugs.

Close menu

Please declare any conflicts of interest you may have with this paper, financial or otherwise.

I do not have a conflict of interest

I would like to declare a conflict of interest

Display my name on the website

Captcha

Renew

I accept the following conditions:

Comments must follow the standards of professional discourse and should focus on the scientific content of the article. Insulting or offensive language, personal attacks and off-topic remarks will not be permitted. Comments must be written in English. Preprints reserves the right to remove comments without notice. Readers who post comments are obliged to declare any competing interests, financial or otherwise.

* All users must log in before leaving a comment

Notify me about updates to this article or when a peer-reviewed version is published.

An Analysis of Prize Money Distributions in Snooker

Abstract

Keywords

Subject

Comments (1)