Article
Version 2
Preserved in Portico This version is not peer-reviewed
Nonlinear Generalisation of Quantum Mechanics
Version 1
: Received: 23 August 2021 / Approved: 27 August 2021 / Online: 27 August 2021 (14:03:12 CEST)
Version 2 : Received: 12 October 2021 / Approved: 14 October 2021 / Online: 14 October 2021 (15:11:22 CEST)
Version 3 : Received: 30 June 2022 / Approved: 1 July 2022 / Online: 1 July 2022 (10:49:24 CEST)
Version 2 : Received: 12 October 2021 / Approved: 14 October 2021 / Online: 14 October 2021 (15:11:22 CEST)
Version 3 : Received: 30 June 2022 / Approved: 1 July 2022 / Online: 1 July 2022 (10:49:24 CEST)
How to cite: Jamali, A. Nonlinear Generalisation of Quantum Mechanics. Preprints 2021, 2021080525. https://doi.org/10.20944/preprints202108.0525.v2 Jamali, A. Nonlinear Generalisation of Quantum Mechanics. Preprints 2021, 2021080525. https://doi.org/10.20944/preprints202108.0525.v2
Abstract
It is known since Madelung that the Schrödinger equation can be thought of as governing the evolution of an incompressible fluid, but the current theory fails to mathematically express this incompressibility in terms of the wavefunction without facing problem. In this paper after showing that the current definition of quantum-mechanical momentum as a linear operator is neither the most general nor a necessary result of the de Broglie hypothesis, a new definition is proposed that can yield both a meaningful mathematical condition for the incompressibility of the Madelung fluid, and nonlinear generalisations of Schrödinger and Klein-Gordon equations. The derived equations satisfy all conditions that are expected from a proper generalisation: simplification to their linear counterparts by a well-defined dynamical condition; Galilean and Lorentz invariance (respectively); and signifying only rays in the Hilbert space.
Keywords
quantum mechanics; foundations of quantum mechanics; nonlinear equation; nonlinear quantum mechanics; nonlinear schrödinger equation
Subject
Physical Sciences, Particle and Field Physics
Copyright: This is an open access article distributed under the Creative Commons Attribution License which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Comments (1)
We encourage comments and feedback from a broad range of readers. See criteria for comments and our Diversity statement.
Leave a public commentSend a private comment to the author(s)
* All users must log in before leaving a comment



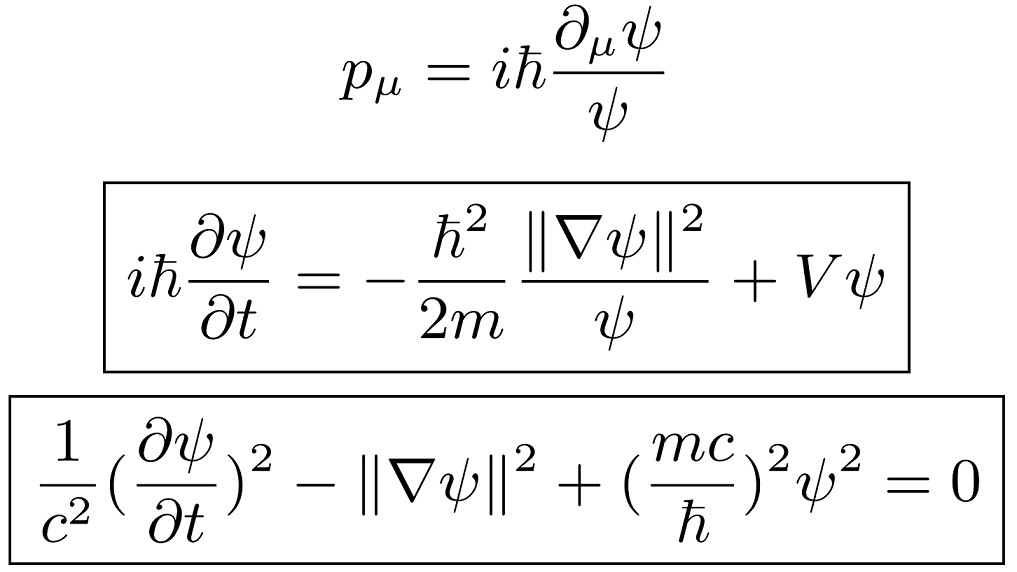





Commenter: Alireza Jamali
Commenter's Conflict of Interests: Author
* The part on Weinberg's Homogeneity condition corrected
* Conclusion section added
* References updated and improved