preprints.org > chemistry and materials science > polymers and plastics > doi: 10.20944/preprints202306.1836.v1

Preprint Article Version 1 Preserved in Portico This version is not peer-reviewed

Version 1 : Received: 26 June 2023 / Approved: 26 June 2023 / Online: 26 June 2023 (21:55:19 CEST)

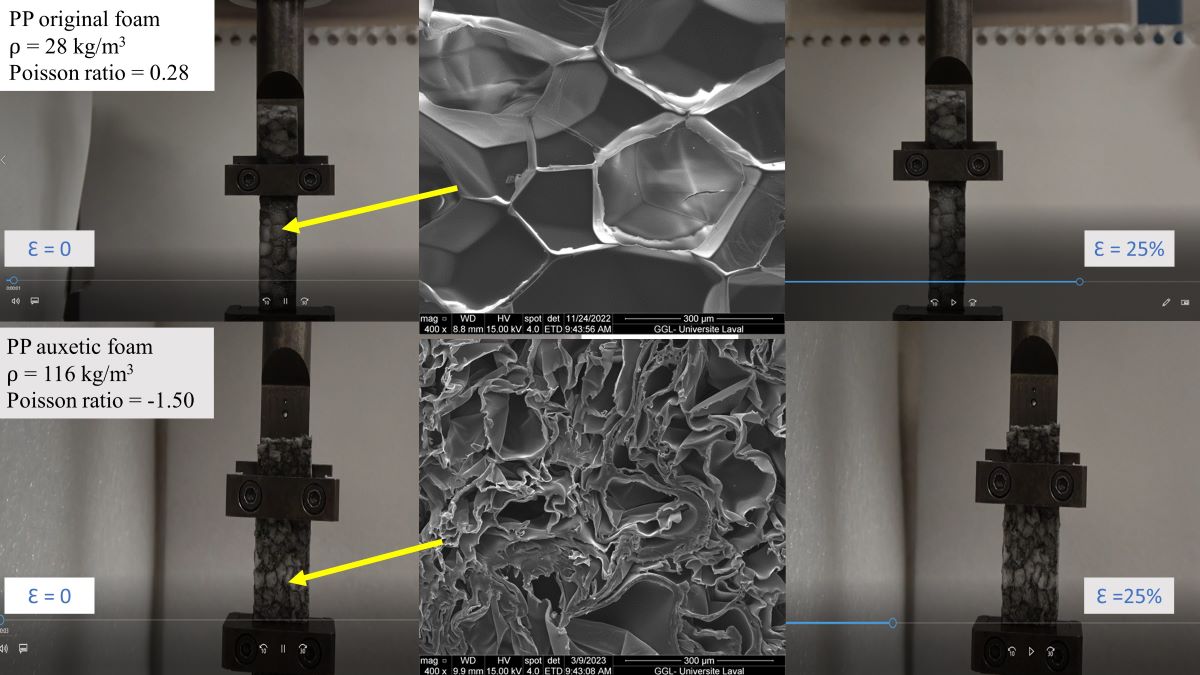
In this work, a simple and environmentally friendly process combining low pressure (vacuum) and mechanical compression is proposed to convert recycled polypropylene (PP) foams (28 kg/m3) into low density foams (90-131 kg/m3) having negative tensile and compressive Poisson's ratios (NPR). The effect of processing conditions (vacuum time, temperature, and mechanical pressure) was studied. Based on the optimized conditions, the tensile Poisson’s ratio of the resulting auxetic foams reached −1.50, while the minimum compressive Poisson's ratios was −0.32 for the same sample. The foam structure was characterized via morphological analysis (SEM) to determine any changes related to the treatment applied. Finally, tensile and compressive properties (Young's modulus, strain energy, energy dissipation and damping capacity) are also presented and discussed. It was observed that the mechanical properties of the resulting auxetic foams were improved compared to the original PP foam (PP-O) for all tensile properties in terms of modulus (19.9 to 59.8 kPa), strength (0.298 to 1.43 kPa) elongation at break (28 to 77%), energy dissipation (14.4 to 56.3 mJ/cm3) and damping capacity (12 to 19%). Nevertheless, improvements were also observed under compression in terms of energy dissipation (1.6 to 3.6 mJ/cm3) and damping capacity (13 to 19%). These auxetic foams can find applications in sport and military protective equipment, as well as any energy mitigation system.

Polypropylene; foam; upcycling; negative Poisson's ratio; auxetic materials; cellular structure; mechanical properties.

Chemistry and Materials Science, Polymers and Plastics

* All users must log in before leaving a comment