Submitted:
07 February 2022
Posted:
08 February 2022
You are already at the latest version
Abstract
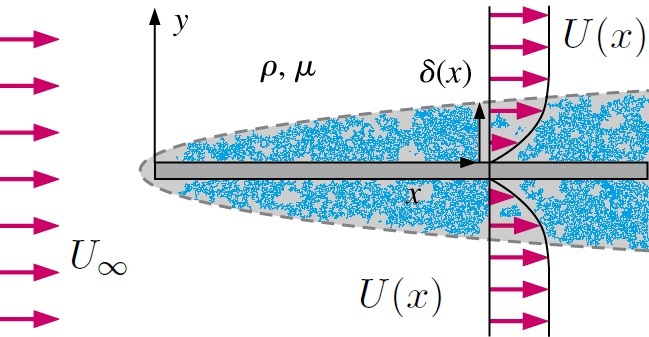
The exact similarity solutions (also called as special exact solutions) of two dimensional laminar boundary layer were obtained by Blasius in 1908, however, no similarity solutions for two dimensional turbulent boundary layers have been reported in the literature. With the help of dimensional analysis and invariance principle, Prandtl mixing length $\ell=\kappa y$ for one dimensional turbulent boundary layer is extended to $\ell(x,y)=\kappa y (1-\frac{y}{\delta})\sqrt{\frac{\nu}{U\delta}} $ for the two dimensional turbulent boundary layers, furthermore, with a similarity transformation, we successfully transform the two dimensional turbulent boundary layers partial differential equations into a single ordinary differential equation $f'''+ ff''+\beta(1-f'^2)+\kappa^2[\eta^2(1-\eta)^2f''|f''|]'=0$. As an application, similarity solutions of the two dimensional turbulent boundary layer on a flat plate at zero incidence have been studied in detail. To solve the ordinary differential equation numerically, a complete Maple code is provided.
Keywords:
Turbulent boundary layers
; laminar boundary layers
; similarity transformation
; similarity solution
; Prandtl mixing length
; Reynolds number
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Downloads
553
Views
306
Comments
0
Subscription
Notify me about updates to this article or when a peer-reviewed version is published.
MDPI Initiatives
Important Links
© 2025 MDPI (Basel, Switzerland) unless otherwise stated




