Solving Prandtl-Blasius Boundary Layer Equation Using Maple

Bohua Sun

doi:10.20944/preprints202008.0296.v2

Preprint Article Version 2 Preserved in Portico This version is not peer-reviewed

Version 1 : Received: 12 August 2020 / Approved: 13 August 2020 / Online: 13 August 2020 (08:32:24 CEST)
Version 2 : Received: 4 September 2020 / Approved: 5 September 2020 / Online: 5 September 2020 (09:33:32 CEST)

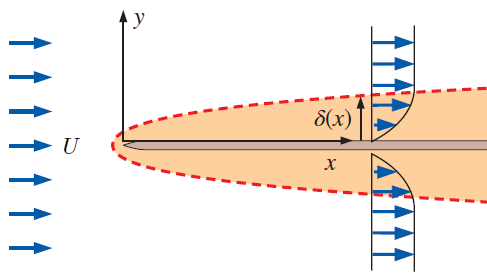
A solution for the Prandtl-Blasius equation is essential to all kinds of boundary layer problems. This paper revisits this classic problem and presents a general Maple code as its numerical solution. The solutions were obtained from the Maple code, using the Runge-Kutta method. The study also considers convergence radius expanding and an approximate analytic solution is proposed by curve fitting. Similarly, the study resolves some boundary layer related problems and provide relevant Maple codes for these.

Prandtl boundary layer; Prandtl-Blasius equation; numerical solution; Runge-Kutta method; Maple

Physical Sciences, Fluids and Plasmas Physics

Comment 1

Received: 5 September 2020
Commenter: Bohua Sun
Commenter's Conflict of Interests: Author

Comment: Add more solutions to Blasius related problems, almost includes all laminar boundary problems are discussed in the book of H. Schlichting and K. Gersten’s book, namely Boundary Layer Theory, 8th ed. 2000.

+ Respond to this comment

Not displayed online.

Please leave your comment to this article below.

Mathematical equations can be typed in either LaTeX formats \\[ ... \\] or $$ ... $$, or MathML format <math> ... </math>. Try the LaTeX or MathML example.

Type equation:

Preview:

Copy equation into message below

Close menu

Please click a symbol to insert it into the message box below:

Please enter the link here:

Optionally, you can enter text that should appear as linked text:

Copy link into message below

Close menu

Please enter or paste the URL to the image here (please only use links to jpg/jpeg, png and gif images):

Copy image into message below

Close menu

Type author name or keywords to filter the list of references in this group (you can add a new citation under Bibliography):

No existing citations in Discussion Group

Wikify editor is a simple editor for wiki-style mark-up. It was written by MDPI for Sciforum in 2014. The rendering of the mark-up is based on Wiky.php with some tweaks. Rendering of mathematical equations is done with MathJax. Please send us a message for support or for reporting bugs.

Close menu

Please declare any conflicts of interest you may have with this paper, financial or otherwise.

I do not have a conflict of interest

I would like to declare a conflict of interest

Display my name on the website

Captcha

Renew

I accept the following conditions:

Comments must follow the standards of professional discourse and should focus on the scientific content of the article. Insulting or offensive language, personal attacks and off-topic remarks will not be permitted. Comments must be written in English. Preprints reserves the right to remove comments without notice. Readers who post comments are obliged to declare any competing interests, financial or otherwise.

* All users must log in before leaving a comment

Notify me about updates to this article or when a peer-reviewed version is published.

Solving Prandtl-Blasius Boundary Layer Equation Using Maple

Abstract

Keywords

Subject

Comments (1)