Zanetti, F.; Bergamaschi, L. Scalable Block Preconditioners for Linearized Navier-Stokes Equations at High Reynolds Number. Algorithms2020, 13, 199.
Zanetti, F.; Bergamaschi, L. Scalable Block Preconditioners for Linearized Navier-Stokes Equations at High Reynolds Number. Algorithms 2020, 13, 199.
Zanetti, F.; Bergamaschi, L. Scalable Block Preconditioners for Linearized Navier-Stokes Equations at High Reynolds Number. Algorithms2020, 13, 199.
Zanetti, F.; Bergamaschi, L. Scalable Block Preconditioners for Linearized Navier-Stokes Equations at High Reynolds Number. Algorithms 2020, 13, 199.
Abstract
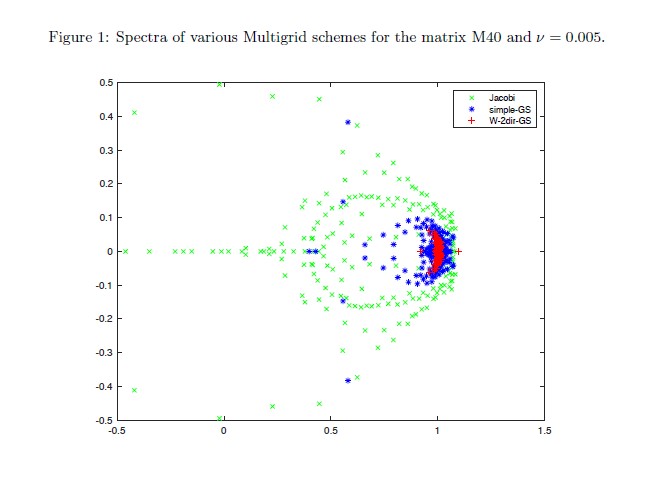
We review a number of preconditioners for the advection-diffusion operator and for the Schur complement matrix which in turn constitute the building blocks for Constraint and Triangular Preconditioners to accelerate the iterative solution of the discretized and linearized Navier-Stokes equations. An intensive numerical testing is performed onto the driven cavity problem with low values of the viscosity coefficient. We devise an efficient multigrid preconditioner for the advection-diffusion matrix which, combined with the commuted BFBt Schur complement approximation, and inserted in a 2 x 2 block preconditioner, provides convergence of the GMRES method in a number of iteration independent of the meshsize for the lowest values of the viscosity parameter. The low-rank acceleration of such preconditioner is also investigated showing its great potential.
Computer Science and Mathematics, Computational Mathematics
Copyright:
This is an open access article distributed under the Creative Commons Attribution License which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
